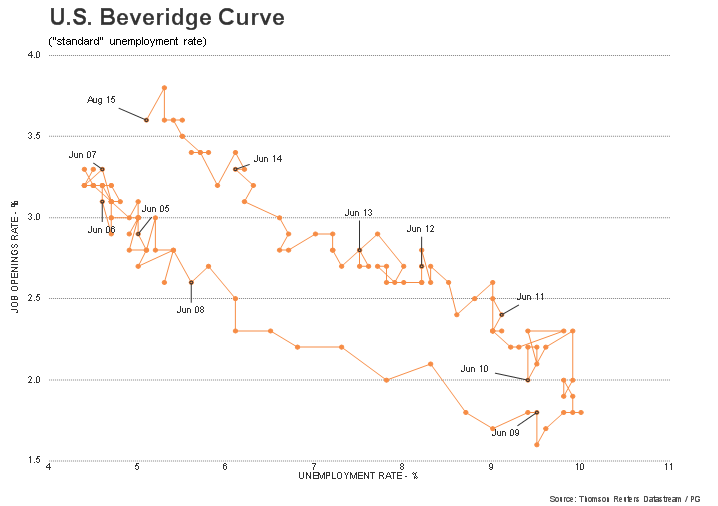
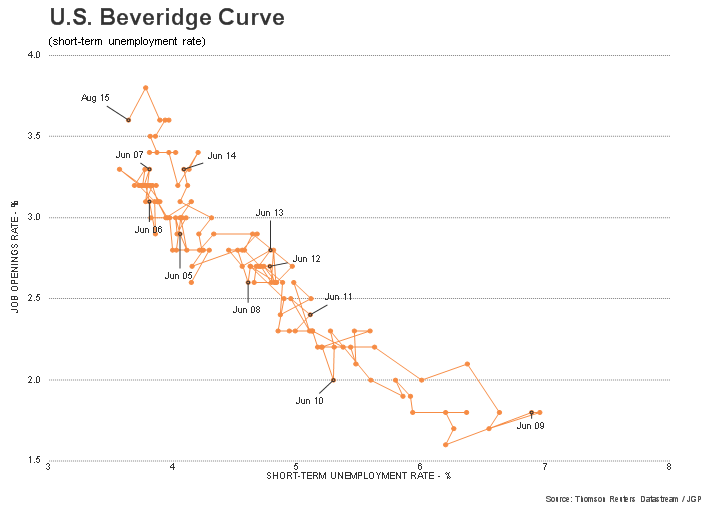
Filtering US GDP noise using CFNAI
Main takeaways:
- “Potential" GDP growth (the one associated with CFNAI at zero) is close to 2.7% using the sample since 1985.
- Latest CFNAI reading of -0.09 in Sep/2015 is usually associated with GDP growth at 2.3%.
Introduction
The Chicago Fed publishes monthly the CFNAI - Chicago Fed National Activity Index, and index designed to gauge overall economic activity and inflation pressure.
The CFNAI is a weighted average of 85 monthly indicators of economic activity, covering four broad categories: production and income; employment; personal consumption & housing; and sales, orders & inventories. A complete list of the variables included can be seen here. The CFNAI is the first principal component (common trend) of the 85 time series.
The CFNAI is constructed to have an average value of zero and a standard deviation of one. A positive reading means the economy is growing above trend. The 3-month moving average of the index is often used to filter monthly noise. According to Chicago Fed research, moving from an expansion period to a reading below -0.7 suggests a recession has begun. A reading above +0.2 suggests the recession has ended, and above +0.7 suggests a period of sustained increasing inflation has begun.
The chart below shows the CFNAI from Mar/1967 to Sep/2015.
The CFNAI has a positive correlation with GDP growth. The chart below shows that GDP trend growth (as measured by a CFNAI at zero) is around 2.8% for the full sample (when based on a linear regression). When looking at a local linear regression (loess) model, GDP trend growth is estimated at 2.7%, and the latest CFNAI printed -0.09 (3mma, Sep/2015) is associated with GDP growth at around 2.5%.
Checking for parameter stability
Formal tests for structural breaks in the relationship between GDP growth and CFNAI (not shown) do not suggest a statistically significant break in the parameters,i.e., the GDP growth rate equivalent to CFNAI at zero can be assumed constant for the whole sample period.
One way to illustrate that is to plot the intercept \(\alpha\) for the rolling linear regression \(GDP_t = \alpha + \beta \times CFNAI_t + \epsilon_t\) starting with the first 10 years of data up to the most recent quarter. The dotted lines represent one standard deviation of the coefficient estimate and show that there’s no structural break in the coefficient \(\alpha\). However, there is a (not statistically significant) jump in the intercept by mid-1980s, which coincides with the period when inflation finally moderated after the oil shock.
Using data since 1985
The chart below plots CFNAI and GDP growth restricting the sample to start in 1985. GDP trend growth (as measured by a CFNAI at zero) is around 2.8% for the sample since 1985 (when based on a linear regression). When looking at a local linear regression (loess) model, GDP trend growth is estimated at 2.7% in the same period. The latest print of CFNAI is associated with GDP growth at around 2.3% (chart).
Using data since 2000
Restricting the sample to start in 2000 we obtain:
GDP trend growth (as measured by a CFNAI at zero) is around 2.5% for the sample since 2000 (when based on a linear regression). When looking at a local linear regression (loess) model, GDP trend growth is estimated at 2.4% in the same period. The latest print of CFNAI is associated with GDP growth at around 2% (chart).
Dr. Paulo Gustavo Grahl, CFA (2015-10-26)